So I was sitting in my office
the other day, and Arnold Schwarzenegger walked in. I was kind of
busy at the time, but, you know, when Arnold walks in you sort of pay attention.
The conversation went something like this:
|

|
Arnold:
HEY MIHOS, there.
|
|
|
Me: Hey
Arnold. How's California?
|
|

|
Arnold:
Screw California. I've got bigger things on my mind. I have some questions for you. I have heard
rumors that there is no dark matter, that it all can be explained by
MOND. That would be good, right, we could kill off
this dark matter stuff?
(<-- shh, Arnold is thinking)
|
|
|
Me: Well,
some people think so.
|
|

|
Arnold:
Well why don't you just run a simulation with that big fancy computer of yours? It should be child's play
-- shouldn't it be just a one line change to your force law?
|
|
|
Me: Well,
it's not that simple.
|
|

|
Arnold:
Do it now! NOW!
|
|
|
Me: OK,
let me explain why it's complicated. N-body simulations represent mass
by individual particles, right?
|
|

|
Arnold:
What?
|
|
|
Me: And
we get the force on a particle by summing up the Newtonian forces from
all the other particles, right?
|
|

|
Arnold:
What?
|
|
|
Me: Well
the standard representation of MOND acceleration as the geometric mean
of the Newtonian acceleration and the MOND characteristic acceleration
means that accelerations doesn't add correctly.
|
|

|
Arnold:
What?
|
|
|
Me: OK,
let's look at it this way. We'll take a particle with mass 4M and calculate
the Newtonian acceleration a test particle at distance r would feel.
|
|

|
Arnold:
Why 4M?
|
|
|
Me: hang
on, just watch. So we calculate the Newtonian acceleration like this:
|
|

|
Arnold:
Yes, I remember that from my freshman physics class.
|
|
|
Me: Good!
OK, now lets break that particle up into 4 smaller particles each of mass
M.
|
|

|
Arnold:
I will break that puny particle with my superstrong muscles.
|
|
|
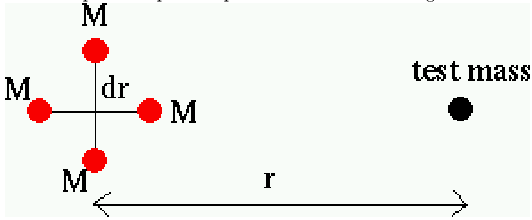
Me: OK,
great, thanks. Now we'll arrange those particles like this:
|
|

|
Arnold:
It looks like two crossed barbells. I must lift them.
|
|
|
Me: No
Arnold, leave them alone. Now we again calculate the Newtonian acceleration
of the test particle.
|
|

|
Arnold:
How?
|
|
|
Me: Just
by summing up the Newtonian forces from individual particles. Like this:
|
|

|
Arnold:
Yah. of course, how silly of me.
|
|
|
Me: which
we can rewrite like this:
where x = dr/r << 1
|
|

|
Arnold:
check, please.
|
|
|
Me: Now
we are going to expand each of these terms ...
|
|

|
Arnold:
You mean like my biceps expand?
|
|
|
Me: ...as
a series, keeping terms to x2:
|
|

|
Arnold:
My head hurts.
|
|
|
Me: Maybe
it's a tumor?
|
|
|
Arnold:
IT'S NOT A TUMOR!
|
|
|
Me: OK,
just checking. Anyway, we can simplify this with algebra. You remember
algebra?
|
|
|
Arnold:
Shut up! - no one
likes a smartass.
|
|
|
Me: So
we get
See, Arnold? We get the same answer.
|
|

|
Arnold:
Hey, very cool, there! You computational astrophysicists are pretty
smart people for punyboys.
|
|
|
Me: Thanks.
OK, now let's do the same thing for the usual parameterization of MOND:
|
|

|
Arnold:
That's a lot of math, there. I do not like so much math. Do not anger
me, punyboy, or I will smash your grandmother
into millions of tiny pieces!
|
|
|
Me: Hang
in there, big fella. So if the original mass is 4M then the acceleration
on the test particle is now:
|
|

|
Arnold:
Can we break up that particle now? I wish to break things.
|
|
|
Me: Be
my guest. We'll do that and add forces again, like this:
|
|

|
Arnold:
This math looks messy. I don't like this.
|
|
|
Me: Don't
worry, we can collect terms
and then do that series thingy again:
|
|

|
Arnold:
Enough! STOP IT!
|
|
|
Me: Arnold,
we're almost there. A little algebra and we get:
Do you see the problem?
|
|

|
Arnold:
That can't be right. Maria, get the car. We're leaving.
|
|
|
Me: Yep,
you've got it. For x<<1, we get
which is twice the original MOND acceleration.
|
|
|
Arnold:
Mihos, you punyboy, explain it to me. Why
is that a problem?
|
|
|
Me: Our
"breaking up the particle" experiment is equivalent to running a simulation
with larger particle number -- i.e., we have more particles of lower mass
to represent the mass. But you've just seen that while that works under
Newtonian gravity, it doesn't work in this representation of MOND. The accelerations
change as you change the particle numbers.
|
|
|
Arnold:
So you mean MOND is wrong?
|
|
|
Me: No,
I mean implementing MOND in this way in N-body simulations -- like many
people seem to be trying to do -- doesn't work. It's not a simple "one
line fix" to the N-body codes. Codes that treat it that way, well, you be
the judge...
|
|
|
Arnold: This
sounds too hard for me. I will go solve California's budget problems instead.
Good luck, Mihos.
|
|
|
Me: Good luck
yourself, Arnold.
|
And so off Arnold went. But questions like his don't go away -- his curiosity
will eventually get the best of him, and he'll be back. Yessiree, I'm sure
of it -- he'll be back.
|