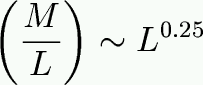Kinematics of Ellipticals
Remember how we characterize velocity of populations
of stars.
-
rotation (v): the net
rotational velocity of a group of stars
-
dispersion (sigma): the
characteristic random velocity of stars
In the disk of our galaxy, v=220 km/s, sigma=30 km/s, so
v/sigma
~ 7. This is called a cold disk.
Elliptical galaxies have much higher velocity dispersions,
100s of km/s. These are kinematically hot
systems. v/sigma ranges (roughly) from 0 to 1. |
|
Would you expect flattened ellipticals to have higher
or lower values of v/sigma? Why?
|
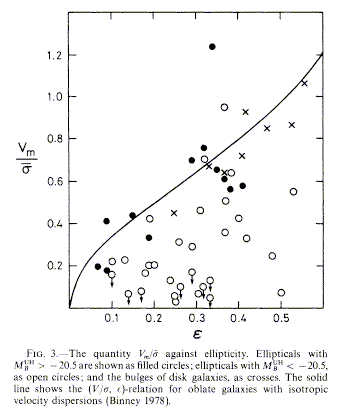
v/sigma actually correlates with luminosity.
-
Lower luminosity ellipticals have higher v/sigma -- rotationally
supported.
-
Higher luminosity ellipticals have lower v/sigma -- pressure
supported. Stars have different characteristic speeds along different axes.
The figure to the right shows v/sigma plotted against
ellipticity (epsilon). The line shows expected shape for rotationally
supported galaxies. (from Davies et al 1983) |
 |
The Faber-Jackson law
Remember the Tully-Fisher law
for disk galaxies: L ~ v4.
Can we make a similar law for elliptical galaxies using luminosity and velocity dispersion?
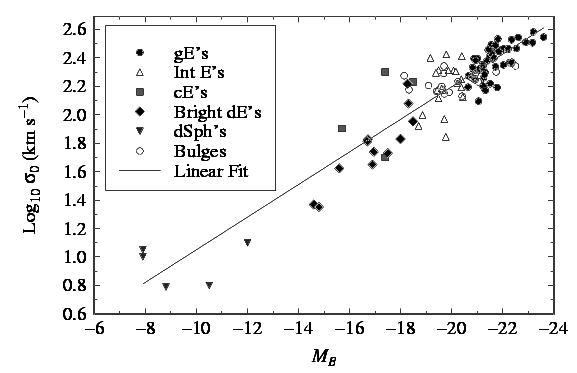
The Faber-Jackson law
has a lot of scatter: at a given velocity
dispersion, there is a range of +/- 2 magnitudes in luminosity. Compare
this to the Tully-Fisher relationship, where at a given circular velocity,
there is a range of a few tenths of a magnitude in luminosity.
Clearly, there is something messing with the relationship
-- a second parameter.
The Fundamental Plane
In 1987, two teams of astronomers identified the second parameter
-- the effective radius. Rather than two parameters correlating (in which
case you fit a line), there are three parameters correlating (in which
case you fit a plane).
We have 4 things we can measure:
-
Luminosity (L)
-
Effective radius (re)
-
Mean surface brightness (<Ie>)
-
Velocity dispersion (sigma)
There are only three independant
variables here (L, re, and <Ie> are
not all independant).
If you plot one versus another, the third introduces scatter,
for example:
Surface brightness versus luminosity
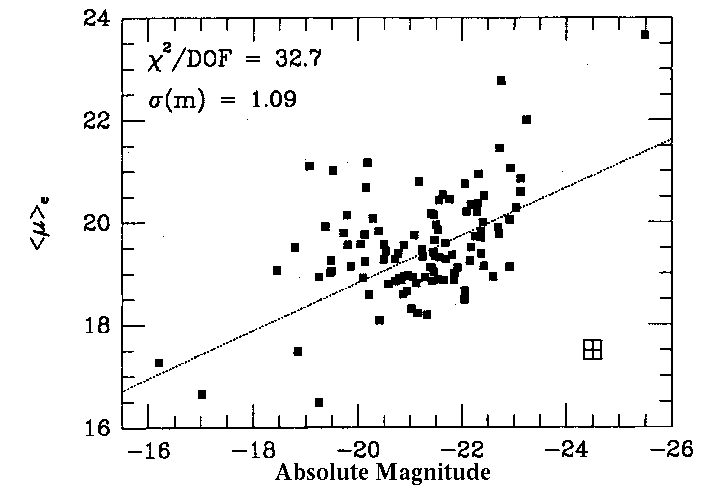
|
Velocity dispersion versus effective radius
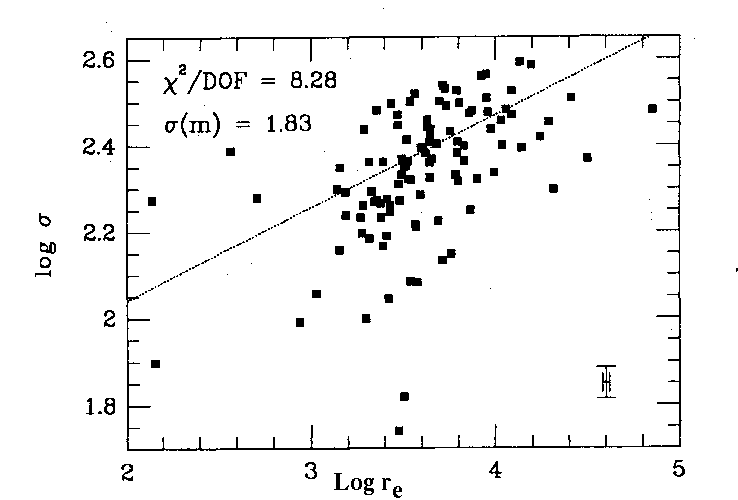
|
But if you plot one versus a combination of the other
two, you can see a very tight correlation:
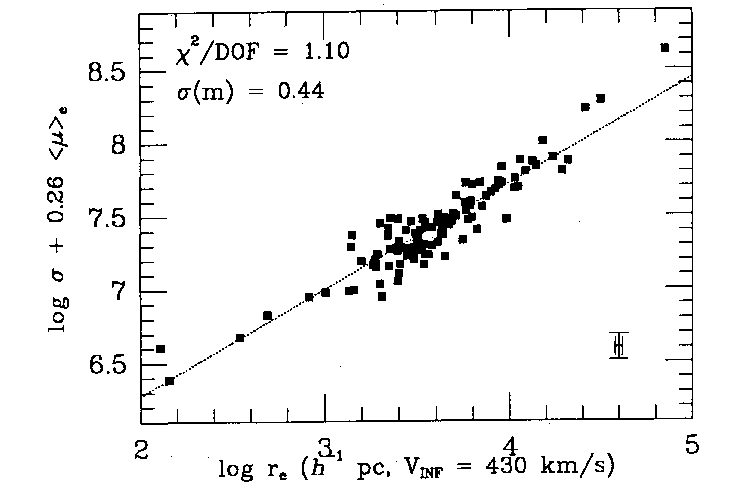
This correlated plane is now referred to as the
fundamental plane. Since we have four observables, only three
of which are independent, there are different representations of the FP
which are all expressing the same thing. Here is another one:
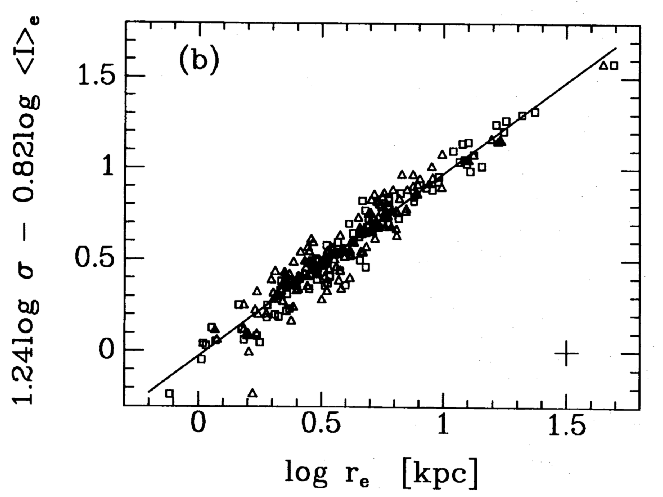
Or in other words
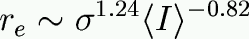
Examples of uses of the Fundamental Plane:
- Distance Measuring: In the FP, re
is measured in physical units: kpc. So if you know the velocity
dispersion and effective surface brightness (both of which are distance
independent measures), you
can use the FP to work out the physical size of the galaxy. If you also
measure its angular size, you can use trigonometry to solve for the
distance to the galaxy.
- Studying Galaxy Structure and Evolution: You can use simple algebra to show that one implication of the fundamental plane is that total (not stellar) mass-to-light ratio depends
on galaxy luminosity:
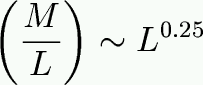
Why would this be?
Whatever model we come up with to explain the formation and evolution
of galaxies must also explain why more luminous galaxies have higher
total mass-to-light ratios.
Central black holes in ellipticals
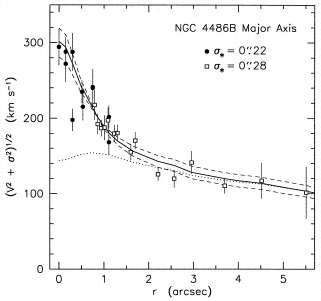
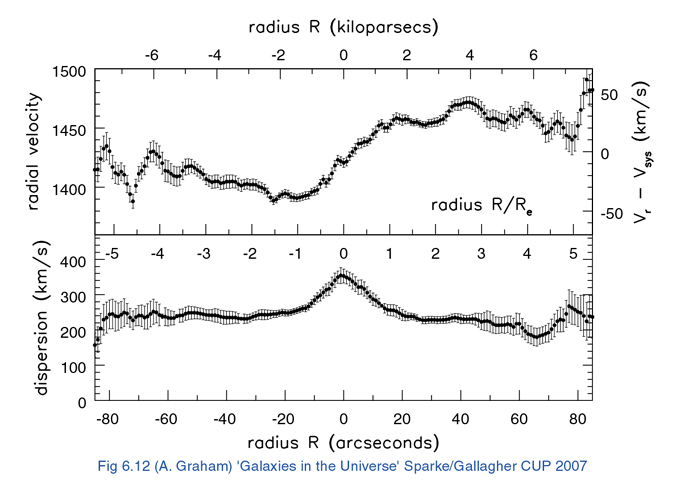
Stellar kinematics in the
cores of nearby ellipticals show a rise in the central velocity
dispersion. The stars are moving too fast for their gravitational
potential -- some "dark mass" must be there.
We can work out the "demographics" of black holes this way and
study their connection to their host galaxies (courtesy Kormendy):
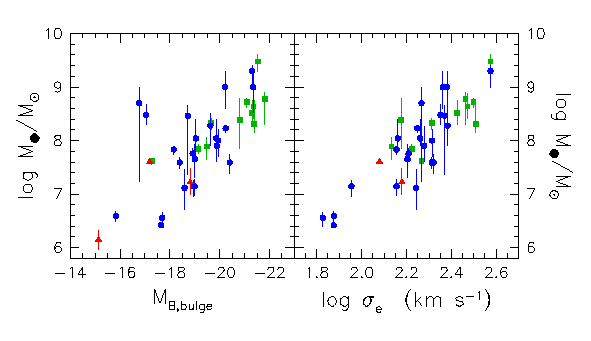
Plotted on the left is the black hole mass vs the absolute
magnitude of the "bulge" of a galaxy, where in this case "bulge" refers
to any spheroidal part of a galaxy -- ie, for a spiral galaxy it is the
stellar bulge, while for an elliptical galaxy it is the entire galaxy.
The implication here is that the black hole mass is about 0.1% of the
bulge mass of a galaxy.
Plotted on the right is the black hole mass vs the velocity
dispersion of the galaxy.